PART I
(June, 2004)
Clearly there will be great difficulties with this subject and consequently there will only be degrees of understanding. Such a model has to have a framework which underlies all existing knowledge and experience, ranging from the God concept to the trivial. It should explain, handle or embrace all fundamental issues, for example:
The reader may wish to study first the author's article Time as an Infinite Fractal, since time manifesting as an infinite fractal arises from the infinite fractal aspect of energy. Note that although the phenomenon of fractals, first evaluated in the 1960s by Benoit Mandelbrot, was considered by some to be the greatest discovery in mathematics, it is even more significant, with far reaching applications.
The heart of the difficulty is that our minds are formatted for linear operations in this third dimension. Moreover when we extend this into nonlinear (inner) perceptions such as in art and music appreciation but also in many underlying concepts to intellectual pursuits, and then attempt to observe this more holistic state of mind, it breaks down (quantum reduces) to the ego-conscious observer reality of the physical sense perceptions (the parts within or representing the whole).
In other words, we can't observe analytically, a state of unity and multiconnected phenomena, since the objective observation process breaks them down into linear parts. It is important to stress how difficult this material is otherwise the point will be missed by many.
If we think of ourselves on a single 2D flat plane, movement and thought along this plane would be what we are calling linear. Nonlinearity would come in from imaginary countless other layers superimposed 'above' our familiar single linear plane. In reality though, it is not 'above' but in expanding spheres (concentric circles in 2D).
In truth each point in our plane is influenced by the upper levels. We are merely introducing the subject by means of simple examples or analogies. In effect the behaviour of a particle or a living entity on the bottom plane is governed ultimately by the whole of the countless layers. This is where the real difficulty arises; to obtain some grasp of the effect of infinitely superimposed nonlinear variables. This is the key problem in understanding the mind's (or universal or nature's) computer system.
Perhaps a very simple analogy depicting only two variables (in fact only one nonlinear variable) would help; that of taking the dog for a walk. The dog's behaviour represents the external view of our material world of physical senses or the first plane. The human (upper plane) represents a higher order of control. The leash links the two together and determines the boundary conditions imposed on the bottom plane (the dog).
The longer the leash, the greater the gap between the levels and the more freedom the dog has. This also means the more probable choices it has relative to the human's control. Moreover, we see that the decisions of the human are superimposed on those of the dog, and the dog's behaviour acts within the context of the human's control. If one could observe only the dog (analogy: external world), its activity could not be understood without taking into account the superimposed human control (higher order, for example, soul level).
This gives the reader an idea of the resultant effect of only two combined variables---the dog's control and the human control. These are each equivalent to fractal levels. Also the dimensional gap between two such fractals determines how much randomness there is between the two fractal levels or how many probabilities there are for selection (choices, freewill).
The longer the leash the more possibilities the dog can experience on its own, but note that it then has less guidance and it is in effect more disconnected. We can refer to the lower level control (the dog's) as local control (LC) and the upper, as global control (GC). LC is always in the context of GC. But this GC becomes local relative to a greater GC. All LC and GC programmes occur at fractal levels.
What would be the most simple structure for the initial development of such a model? It would be a circle. Let us now elaborate on this circle and make it into a spiral; a little like connecting together many circles---see Figure 1. This should be imagined as a fine spiral with no gaps (beyond about quantum size).
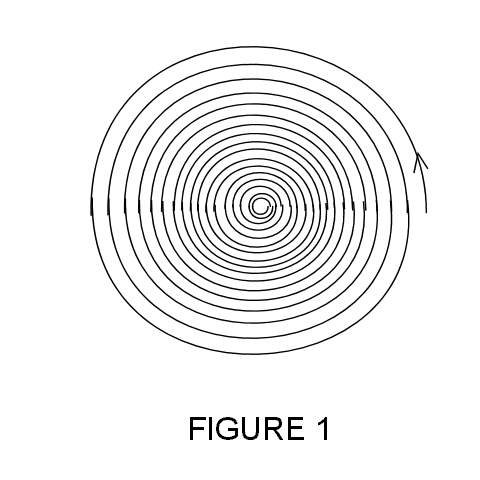In 2D they are like circles within circles but in 3D or more they are like shells within shells connected as a total spiral (ideally this should then be envisaged in 4D to give perfect super-symmetry when we introduce the anti-spiral). This is the same as the vortex model given in other articles where much more detail is presented, such as the article, The Basic Energy Unit ---which, in particular, shows the higher-dimensional nature of the vortex.
In terms of three or more dimensions, this spiral would resemble spheres within spheres. The direction of motion of the energy is inwards from be periphery to the centre. This is essentially a model for a vortex. Current science interprets reality at the particle level and thus focusses only on the external world and the lowest contextual orders of the spiral near the centre.
What logical justification do we have for introducing these concentric circles or spirals? The spiral or vortex is a natural and efficient way for energy to transduce from higher dimensions to low. The reader is referred to the articles, The Source of Fractals and The Theory of One.
Returning to Figure 1, what may be surprising now is that each turn of the spiral (a circle) is a potential fractal level. Figure 2 shows three potential fractal levels highlighted---meaning they have manifested a form into reality. If we imagine an opposite total spiral underlying the one in Figure 1---see Figure 3, a 'side' view---using the 2D or 3D view of this, we can give it standing wave structures at each of the levels where the opposite interacts. (There are no stable forms or even time without counteraction and limitations.)
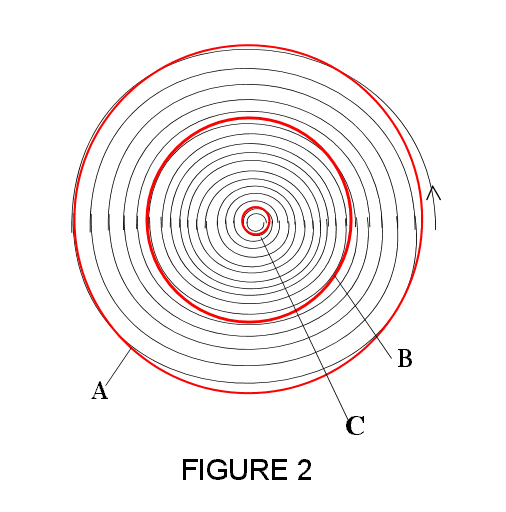
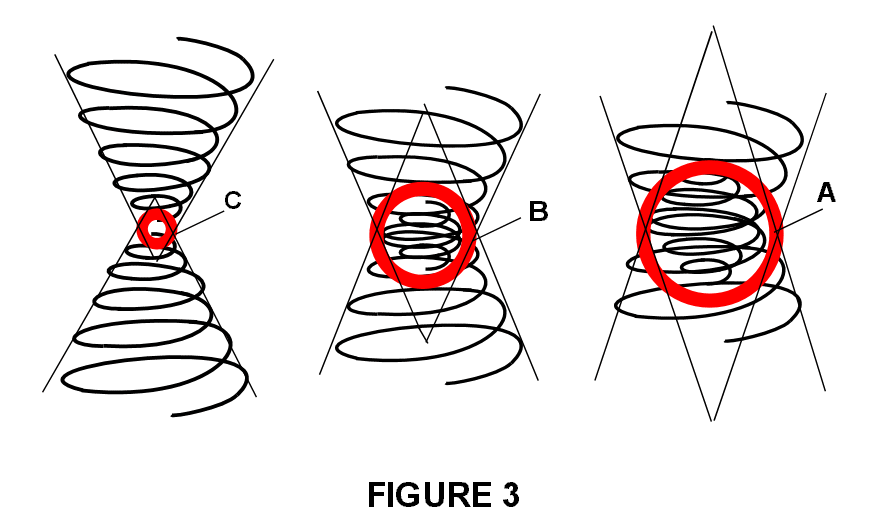
Figure 2 shows the three fractal shells formed by opposing spirals, creating standing waves. Figure 3 gives an idea of the inner structure. Since this has been covered in other articles we will not repeat the explanation as we wish to move on to programming applications to nature and the universe.
In effect, we are describing a vortex matrix from which all forms emanate. In Figure 2 the fractal strata A, B, C could be a universe, a planet, an atom, respectively. One might see at this point that the universe, circle A will spiral through other fractal levels, not shown: into many galaxies, which in turn spiral into many star systems, which in turn spiral into planets, such as fractal level B in Figure 2, and further eventually into atoms, say, fractal C (see later figures).
These must be stable stationary states---and there will be many in reality. Note that the spiral forms closed systems at these fractal levels (which on the negative side provide a mechanism of entrapment and evolutionary control).
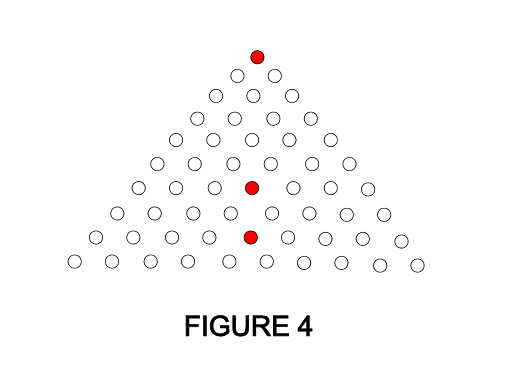Figure 4 gives another view of this. The top represents the unity of everything below it, and corresponds to A. Then B and C are levels further down.
Figure 4 gives another view of this. The top represents the unity of everything below it, and corresponds to A. Then B and C are levels further down.
Figure 3 shows the connection between the spiral and the anti-spiral, and is a 'side' view of Figure 2. The next feature we need to mention is that each spiral circle in Figure 2 functions in the context of the next higher (larger circle) context. For example the fractal level B, say, a planet, will regulate the order of the lower fractal level of atoms at C. Also the dimensions and frequencies increase towards the greater spiral circles.
The significance and meaning of dimensions and frequencies is not easy, except to recognize that the probabilities increase with the widening spiral, that is, greater degrees of freedom, greater number of choices, increased variety, etc. The centre circle of the spiral (Figure 3) would represent a particle or a world made up of these units; this centre circle is the manifestation region of the interacting spirals and can be any level such as A, B, or C, where stable standing waves are formed.
So far then we have one big spiral or vortex (with its opposite), which splits up into a myriad of smaller ones---we need many smaller circles to make up a reality. When a split occurs, this forms new fractal levels of stability or existence---the atoms, the planet, universe, in Figure 2. Figure 5 illustrates the multiplication of these spiral fractals---anti-spirals not shown---and can be compared with Figure 4.
An upper fractal contains the innate programming for ordering a lower one, such as Earth and an atom, respectively. We shall occasionally use the analogy of the pyramid company organization, such as the manager being a higher fractal, giving instructions to a ground-floor worker, a lower fractal. The ground-floor worker, manager, executive, president, would represent stable fractals such as in a fern and its fronds, or branches on a tree.
Between and within these fractal levels are the probabilities---the random area. Prior to the advent of chaos theory (and to some degree quantum mechanics) scientists thought that the universe was created from a random condition. This would correspond to the spiral in Figure 1---containing no orders and thus there would really be no fractals and therefore no existence---there would only be the particle level. But science tells us the universe is made up of particles only; however, this is not so. Established fractal levels, such as a planet, galaxy, etc. are also basic, besides elementary particles.
Randomness and unpredictability are essential though to free will and creativity. The random areas are the ones with flexibility and instabilities, available for further programming. The degree of free play between fractals is proportional to the gap between adjacent global control fractals and local control fractals. But even this relation is governed by its context and application. (It is not essential that the following more mathematical analysis is understood.)
If we are applying it to consciousness then it would appear we are saying that the greater the gap between, say, the soul level and its human extension, the more free will the human has. But this applies only when the soul level has set a programme for the human---the soul is free of this program. If the gap was very small the human would act as one with the soul more frequently, thus having still greater freedom. Compare the dog's leash decreasing so that eventually the dog's path is the same as the dog's owner's, with the freedom that the owner has. (Note: 'human' here, is not to be confused with the dog's owner in the analogy.)
Thus there are two counter variables when we increase the gap. If there is a higher programme:
1) the human (soul extension, not the dog analogy) has more freedom since there are more probabilities available 'around' the programme (the dog has a longer leash) and the human can, for instance, try some negative, egotistical paths that are not in the programme,2) but as we increase the gap, the effect of the programme becomes more compulsive (enforced) and not within the choice of the human---it is more unconscious. As we decrease the gap, the programme is more willingly applied by the human, since it is acting more as the soul (oneness with the soul).
The fractal levels are programmes of information but will have boundary conditions that give some free play. For example, a tree might be fixed to have seven fractal levels:
1) trunk,2) first large branch,3) smaller branches . . . still smaller branches to 7) twigs.
However, the fixed programme does not say the trunk and the branches must be of exact thicknesses, or that a branch must begin precisely at such and such a point, or be of such a length before another offshoot branch forms.
There is thus growth creativity in addition to growth programming, according to environmental circumstances. For example, the DNA of a seed or plant will be in continuous communication with higher fractal collective levels for this species, making selections, within boundary conditions, for the next cell placement in its growth.
If the programmer is looking for instructions/programming of the type used in man's artificial computers he won't find them. Man's encoding systems are relative and arbitrary and essentially are governed by algebra formulae, which means representations; let this or that equal whatever. Nature's universal system must be absolute and ubiquitous for all entities and will thus operate on geometric intelligence.
Another simple analogy is to consider a wire net, which has square openings between the wires. The wires give the boundary condition, the dimensional framework for free play and exploration within the squares. However, we have to imagine many nets of different size openings (fine to coarse) all superimposed but hierarchically, as are the circles in Figure 1, or as in a company organization.
The ground-floor worker functions in narrow boundary conditions, the managers have a wider span of operation and the president would be like a 'net' with just one big square opening (spanning all the company activities).
Now, in the human body, for example, arm movements, we have fractals at the fixed points: shoulder, elbow, wrist, fingers. The same basic model applies here. The shoulder movement contains the larger spiral which is a vortex (working with the muscles and regulating the angle of the joints), and the elbow fractal is within this, and so on to the fingers. The fingers operate in the context of the wrist, the wrist in the context of the elbow, etc. See article Free will and Fractals and articles on physical mobility.
This is an efficient system. There could be fractals between shoulder and elbow or elbow and wrist, that is, more joints. In fact there could be continuous fractals (joints) along the arm to produce a prehensile arm, as in a monkey's tail, but the human arm arrangement is considered adequate. We programme the learning patterns for control of angular movements of fingers, wrist, elbow, and shoulder.
Psychologists some 30 years ago discovered experimentally that learning at the shoulder would pass down the ranking system to low levels (fractals) such as elbow, wrist, fingers (previously explained in detail). But learning pattern information would not pass up the rank (hierarchical system from fingers to wrist, elbow, and shoulder). Thus any 'circle' in Figure 1 operates in the context of any greater circle.
And we are not using an analogy when we say that the shoulder vortex passes down its information to a smaller elbow joint vortex, and further to smaller vortices, wrist and fingers, when a sequence of movements is learned. We are actually stating that storage of information for, say, a keyboard sequence of movements comes from the shoulder, where the programme is in a higher-order format and quantum reduces through the lower context and fractals of elbow and wrist, to the fingers. See articles on physical mobility.
Let us now consider another application of these same fractal nonlinear programming principles. This particular example will be used intermittently throughout the text for clarification purposes. Each analogy gives a different angle on the problem for aiding overall understanding.
Consider a soccer team; though this will be too complex for a simple example of the way nonlinear programming works. Therefore let us take the following components only: a goal post, goal keeper, one defense, and one opponent, or offense/attacker. This example is only an analogy to show the mechanics of this computer system since, in particular, the players will have free will (within certain higher-dimensional limits) and be independent of a weak collective effect---compared with the tree, which has a much more formatted programme.
Since we consider that the energy (of creation or fundamental programming)---and this is an inherent part of this system---flows from a large 'circle' to a small one, then for illustration purposes we can put in a programme at level A---see Figure 6. We set the programme at A to give player at D, the attacker, a goal. Note that this is relevant to anyone setting personal goals.
They might activate the goal postulate at this higher order level (many other factors must agree though). Nevertheless we are taking an imaginary case here. Thus the programme has the instruction that a goal is made in, say, a few minutes---because of the free play between fractal levels this interval is quite flexible.
Let's give the attacker the ball. He basically has countless probabilities to choose from as an independent entity. But this is now a programme and all component parts must be interrelated; his probable choices will be less. The individual mode corresponds to the centre of Figure 1---like a particle.
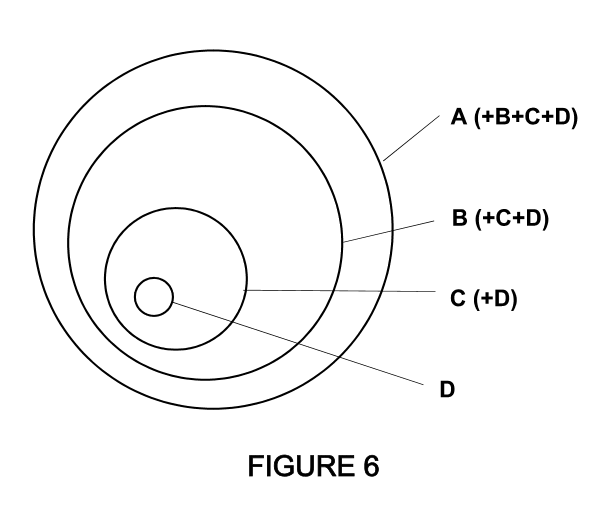In Figure 6 we see that A is the 'president' component in the programme which spans all factors or lower fractal levels. We have in fact already created a fractal postulate by establishing the goal to be achieved.
Figure 6 has been simplified even beyond our three players and the goal post by reducing the programme to only four fractal levels A, B, C, D, but in general there would be a gradient from A to D. This gradient involves the mind and physical processes of the components of the programme.
Returning to the offensive player with the ball. Although he has a complete array of probabilities, choices to select from, such as move right, move left, etc., all these selections will not give the goal because there is a defense and a goal keeper. Nevertheless D has numerous possibilities that will lead to the goal which A will emphasize.
Thus we shift now from fractal control at level D to fractal level C, which spans both defense and attack (keep in mind we are explaining these, one after the other, D, C, B, A, but they are simultaneous and that there is really a gradient going back to total control at A).
Level C's probabilities, which also must lead to the goal, will limit the attack player's choices still further. But this player will make one of these goal selections (since levels B and A are operating, giving simultaneous guidance with a necessary 'homing in' gradient to the goal). We see that the available probabilities are reducing---not in time though; it is simultaneous activity.
Let the offensive player now select a movement, say, to the right for passing the defense. But all the while this is occurring, in addition to A, which is influencing the whole scene, fractal programme component B is functioning and influencing C and D. We may see that B covers the collective interrelationship between offense, defense, and goal keeper, that is, C and D.
Beware of thinking in a sequence, but the material level must play off in time. A learning pattern in skills such as playing a sequence of movements on a keyboard will span a couple of seconds (or so) by the 'president' level, such as fractal A, with a gradient breakdown to D; and a sequence in time is achieved in precisely the same manner as described above. If the reader becomes lost on this nonlinearity/simultaneous concept, go back to the example of the person taking a dog, which is on a long leash, for a walk.
The dog can make decisions where to go within the boundary conditions (context) of the human and leash, but also the human's decisions, such as where to go, are superimposed on this for the final outcome or result.
We specially constructed the soccer example so that it would justify about four fractal levels (which could be understood) but in truth one should realize that, in general, our programme must handle conceptual as well as physically-fixed fractal examples, such as in the case of a tree (branches to twigs), and the full gradient (Figure 1) must be taken into account. Why is this? Why can't we have subprogrammes at A, B, C, D with gaps between as illustrated in this simplified example.
This is because continuous (contiguous) nonlinearity is required, otherwise during those gaps the sequence will have to be predictable linear paths (because of the enforced programme) with no free will or interference occurring, such as the players falling down. The gaps would be acceptable if they could be rigid like the arm arrangement. This is a programme not designed to have steps in it like the arm and its joints; the programme must include the viewpoint of the other players and environmental factors.
In the natural condition of this soccer example there would of course be no head programmer setting a goal to be made, and our example is essentially fictitious for our level of evolution.. However, note that the players, with sufficient cohesion of their minds, could quantum regenerate a goal to be made for their collective group. This means a fractal level at A is now programmed with the goal result.
It means level A will now superimpose on level D at all times, influencing what level D produces or selects in the way of probabilities available to it. Level A would restrict the choices at level D to probabilities that culminate in the goal. This would be controlled through sub-levels B and C.
A programme must contain a complete number of fractal steps according to its requirements and applications. The above fractal strata has been compared with the arm action: A (shoulder), B (elbow), C (wrist), D (fingers). But with the arm there is rigidity between the fractal levels (forearm, upper arm) and programmed fractal levels cannot be added (new joints). Whereas this is flexible in the soccer example, and as stated must therefore have a continuous gradient available.
Note that when we refer to 'linear' we mean only one of the levels would be present, which would be D for us---the material expression---and then a string of Ds would be a linear programme (as in our computers). Life and the universe never work this way; they are always nonlinear, as scientists are beginning to realize.
The reason is that consciousness is inherently multidimensional---a slice of this 'thickness' would be a limited and 'artificial' viewpoint which is what we have in this 3D. Let us continue with further analogies and applications to consciousness in Part II, eventually examining the locomotion aspects of the energies and physical fractal expressions.
Back to Contents (The link leads to further information on the above Paradigm)
The author of the above is Noel Huntley
Noel Huntley, Ph.D.
About the author
Primarily a truth seeker, pushing out the frontiers of knowing has been a lifelong passion of Dr. Huntley; even taking precedence over his remarkable talents as a painter and musician.
Primarily a truth seeker, pushing out the frontiers of knowing has been a lifelong passion of Dr. Huntley; even taking precedence over his remarkable talents as a painter and musician.
Amongst many areas of extensive study, Noel Huntley has investigated UFO and ET reports, chanspiritual phenomena. With a background in physics from Leeds University and formerly employed at the Atomic Weapons Research Establishment, Aldermaston, he later acquired further doctorates externally in psychology and parapsychology and has developed the foundations for a higher-dimensional physics that embraces life, mind, the universe, and the spirit.
This, he considers, will reconcile physics and religion into a spiritual science, in addition to aligning the viewpoints of the atheist and the religionist. While a dedicated scientist, he dares to cross the line between objective investigation and subjective investigation. His contention being that scientific investigation must not be confined to the material and that which is exterior to self.
This, he considers, will reconcile physics and religion into a spiritual science, in addition to aligning the viewpoints of the atheist and the religionist. While a dedicated scientist, he dares to cross the line between objective investigation and subjective investigation. His contention being that scientific investigation must not be confined to the material and that which is exterior to self.
He has written technical manuals and numerous books, articles and papers. Studies also included many other subjects, attaining qualifications in art, music, metaphysics and computers. In addition, research has been conducted in the field of physical mobility, coordination and skills with an evaluation of the true scientific principles involved.
Currently he authors a website which addresses a wide variety of subjects pertinent to the nature of consciousness, ascension and evolution of the individual and our civilization.
Currently he authors a website which addresses a wide variety of subjects pertinent to the nature of consciousness, ascension and evolution of the individual and our civilization.
neling and
No comments:
Post a Comment